Transformer:Regulation of a Transformer

By -

Regulation of a Transformer

1. When a transformer is loaded with a constant primary voltage, the secondary voltage decreases* because of its internal resistance and leakage reactance.

Let                  0V2 = secondary terminal voltage at no-load.

= E2 = EK1 = K V1 because at no-load the impedance drop is negligible.

V2 = secondary terminal voltage on full-load.

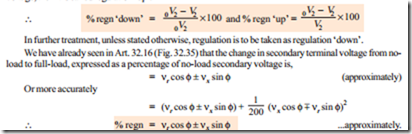
The change in secondary terminal voltage from no-load to full-load is = 0V2 - V2. This change divided by 0V2 is known as regulation ‘down’. If this change is divided by V2, i.e., full-load secondary terminal voltage, then it is called regulation ‘up’.

image

The lesser this value, the better the transformer, because a good transformer should keep its secondary terminal voltage as constant as possible under all conditions of load.

(2) The regulation may also be explained in terms of primary values.

In Fig. 32.51 (a) the approximate equivalent circuit of a transformer is shown and in Fig. 32.51 (b), (c) and (d) the vector diagrams corresponding to different power factors are shown.

The secondary no-load terminal voltage as referred to primary is E¢2 = E2/K = E1 = V 1 and if the secondary full-load voltage as referred to primary is V 2¢ (= V 2/K) then

image

As the transformer is loaded, the secondary terminal voltage falls (for a lagging p.f.). Hence, to keep the output voltage constant, the primary voltage must be increased. The rise in primary voltage required to maintain rated output voltage from no-load to full-load at a given power factor expressed as percentage of rated primary voltage gives the regulation of the transformer.

Suppose primary voltage has to be raised from its rated value V1 to V1¢, then

image

Example 32.43. A-100 kVA transformer has 400 turns on the primary and 80 turns on the secondary. The primary and secondary resistances are 0.3 W and 0.01 W respectively and the corresponding leakage reactances are 1.1 and 0.035 W respectively. The supply voltage is 2200 V. Calculate (i) equivalent impedance referred to primary and (ii) the voltage regulation and the sec- ondary terminal voltage for full load having a power factor of 0.8 leading.

(Elect. Machines, A.M.I.E. Sec. B, 1989)
image
image
 
image
image
Example 32.50. Resistances and Leakage reactance of 10 kVA, 50 Hz, 2300/230 V single phase distribution transformer are r1 = 3.96 ohms, r2 = 0.0396 ohms, x1 = 15.8 ohms, x2 = 0.158 ohm. Subscript 1 refers to HV and 2 to LV winding (a) transformer delivers rated kVA at 0.8 p.f. Lagging to a load on the L.V. side. Find the H.V. side voltage necessary to maintain 230 V across Load- terminals. Also find percentage voltage regulation. (b) Find the power-factor of the rated load- current at which the voltage regulation will be zero, hence find the H.V. side voltage.
(Nagpur University, November 1997)

Solution. (a) Rated current on L.V. side = 10,000/230 = 43.5 A. Let the total resistance and total leakage reactance be referred to L.V. side. Finally, the required H.V. side voltage can be worked out after transformation.

image

For purpose of calculation of voltage-magnitudes, approximate formula for voltage regulation can be used. For the present case of 0.8 lagging p.f.

image

image

Comments

Post a Comment

Popular posts from this blog

Total Loss in a D.C. Generator

By -
Image
Total Loss in a D.C. Generator The various losses occurring in a generator can be sub-divided as follows : where R a = resistance of armature and interpoles and series field winding etc. This loss is about 30 to 40% of full-load losses. Field copper loss. In the case of shunt generators, it is practically constant and I 2 R (or V I sh ). In the case of series generator, it is = I s e 2 R s e where R s e is resistance of the series field winding. This loss is about 20 to 30% of F.L. losses. (iii) The loss due to brush contact resistance. It is usually included in the armature copper loss. (b) Magnetic Losses (also known as iron or core losses), (i) hysteresis loss, W h µ B max f and ( ii ) eddy current loss, W e µ B max f These losses are practically constant for shunt and compound-wound generators, because in their case, field current is approximately constant. Both these losses total up to about 20 to 30% of F.L. losses. (c) Mechanica l Losses. These consist of :...
Read more

Transformer,three phase:Wye/Delta or Y/∆ Connection and Delta/Wye or ∆/Y Connection.

By -
Image
Wye/Delta or Y/∆  Connection The main use of this connection is at the substation end of the transmission line where the voltage is to be stepped down. The primary winding is Y -connected with grounded neutral as shown in Fig. 33.6. The ratio between the secondary and primary line voltage is 1/ 3 times the transformation ratio of each trans- former. There is a 30° shift between the primary and secondary line voltages which means that a Y - D transformer bank cannot be paralleled with either a Y - Y or a D - D bank. Also, third harmonic currents flows in the D to provide a sinusoidal flux. Delta/Wye or ∆/Y Connection This connection is generally employed where it is necessary to step up the voltage as for example, at the beginning of high tension transmission system. The connection is shown in Fig. 33.7. The neutral of the secondary is grounded for providing 3-phase 4-wire service. In recent years, this connection has gained considerable popularity because it can be used to s...
Read more

Testing of d.c.Machines:Brake Test

By -
Image
Brake Test It is a direct method and consists of applying a brake to a water-cooled pulley mounted on the motor shaft as shown in Fig. 31.1. The brake band is fixed with the help of wooden blocks gripping the pulley. One end of the band is fixed to earth via a spring balance S and the other is connected to a suspended weight W 1. The motor is running and the load on the motor is adjusted till it carries its full load current. The simple brake test described above can be used for small motors only, because in the case of large motors, it is difficult to dissipate the large amount of heat generated at the brake. Another simple method of measuring motor output is by the use of poney brake one form of which is shown in Fig. 31.2 ( a ). A rope is wound round the pulley and its two ends are attached to two spring balances S 1 and S 2. The tension of the rope can be adjusted with the help of swivels. Obviously, the force acting tangentially on the pulley is equal to the differ ence ...
Read more