D.c. Motor:Speed of a D.C. Motor
Speed of a D.C. Motor
From the voltage equation of a motor (Art. 27.4), we get
Speed Regulation
The term speed regulation refers to the change in speed of a motor with change in applied load torque, other conditions remaining constant. By change in speed here is meant the change which occurs under these conditions due to inherent properties of the motor itself and not those changes which are affected through manipulation of rheostats or other speed-controlling devices.The speed regulation is defined as the change in speed when the load on the motor is reduced from rated value to zero, expressed as percent of the rated load speed.
Torque and Speed of a D.C. Motor
It will be proved that though torque of a motor is admittedly a function of flux and armature current, yet it is independent of speed. In fact, it is the speed which depends on torque and not vice- versa. It has been proved earlier that
It is seen from above that increase in flux would decrease the speed but increase the armature torque. It cannot be so because torque always tends to produce rotation. If torque increases, motor speed must increase rather than decrease. The apparent inconsistency between the above two equations can be reconciled in the following way :
Suppose that the flux of a motor is decreased by decreasing the field current. Then, following sequence of events take place :
1. Back e.m.f. Eb (= NF/K) drops instantly (the speed remains constant because of inertia of the heavy armature).
2. Due to decrease in Eb, Ia is increased because Ia = (V - Eb)/Ra. Moreover, a small reduction in flux produces a proportionately large increase in armature current.
3. Hence, the equation Ta µ FIa, a small decrease in f is more than counterbalanced by a large increase in Ia with the result that there is a net increase in Ta.
4. This increase in Ta produces an increase in motor speed.
It is seen from above that with the applied voltage V held constant, motor speed varies inversely as the flux. However, it is possible to increase flux and, at the same time, increase the speed provided Ia is held constant as is actually done in a d.c. servomotor.
Example 29.20. A 4-pole series motor has 944 wave-connected armature conductors. At a certain load, the flux per pole is 34.6 mWb and the total mechanical torque developed is 209 N-m. Calculate the line current taken by the motor and the speed at which it will run with an applied voltage of 500 V. Total motor resistance is 3 ohm.
Now, speed may be found either by using the relation for Eb or Ta as given in Art.
Example 29.21. A 250-V shunt motor runs at 1000 r.p.m. at no-load and takes 8A. The total armature and shunt field resistances are respectively 0.2 W and 250 W. Calculate the speed when loaded and taking 50 A. Assume the flux to be constant. (Elect. Engg. A.M.Ae. S.I. June 1991)
Example 29.22. A d.c. series motor operates at 800 r.p.m. with a line current of 100 A from 230-V mains. Its armature circuit resistance is 0.15 W and its field resistance 0.1 W. Find the speed at which the motor runs at a line current of 25 A, assuming that the flux at this current is 45 per cent of the flux at 100 A. (Electrical Machinery - I, Banglore Univ. 1986)
Example 29.23. A 230-V d.c. shunt motor has an armature resistance of 0.5 W and field resistance of 115 W. At no load, the speed is 1,200 r.p.m. and the armature current 2.5 A. On application of rated load, the speed drops to 1,120 r.p.m. Determine the line current and power input when the motor delivers rated load. (Elect. Technology, Kerala Univ. 1988)
Example 29.24. A belt-driven 100-kW, shunt generator running at 300 r.p.m. on 220-V bus- bars continues to run as a motor when the belt breaks, then taking 10 kW. What will be its speed ? Given armature resistance = 0.025 W, field resistance = 60 W and contact drop under each brush = 1 V, Ignore armature reaction. (Elect. Machines (E-3) AMIE Sec.C Winter 1991)
Example 29.25. A d.c. shunt machine generates 250-V on open circuit at 1000 r.p.m. Effective armature resistance is 0.5 W, field resistance is 250 W, input to machine running as a motor on no- load is 4 A at 250 V. Calculate speed of machine as a motor taking 40 A at 250 V. Armature reaction weakens field by 4%. (Electrical Machines-I, Gujarat Univ. 1987)
Example 29.26. A 250-V shunt motor giving 14.92 kW at 1000 r.p.m. takes an armature current of 75 A. The armature resistance is 0.25 ohm and the load torque remains constant. If the flux is reduced by 20 percent of its normal value before the speed changes, find the instantaneous value of the armature current and the torque. Determine the final value of the armature current and speed.
(Elect. Engg. AMIETE (New Scheme) 1990)
Example 29.27. A 220-V, d.c. shunt motor takes 4 A at no-load when running at 700 r.p.m. The field resistance is 100 W. The resistance of armature at standstill gives a drop of 6 volts across armature terminals when 10 A were passed through it. Calculate (a) speed on load (b) torque in N-m and (c) efficiency. The normal input of the motor is 8 kW.
(Electrotechnics-II; M.S. Univ. Baroda 1988)
Tutorial Problems 29.2
1. Calculate the torque in newton-metre developed by a 440-V d.c. motor having an armature resistance of 0.25 W and running at 750 r.p.m. when taking a current of 60 A. [325 N-m]
2. A 4-pole, lap-connected d.c. motor has 576 conductors and draws an armature current of 10 A. If the flux per pole is 0.02 Wb, calculate the armature torque developed. [18.3 N-m]
3. (a) A d.c. shunt machine has armature and field resistances of 0.025 W and 80 W respectively. When connected to constant 400-V bus-bars and driven as a generator at 450 r.p.m., it delivers 120 kW. Calculate its speed when running as a motor and absorbing 120 kW from the same bus-bars.
(b) Deduce the direction of rotation of this machine when it is working as a motor assuming a clockwise rotation as a generator. [(a) 435 r.p.m. (b) Clockwise]
4. The armature current of a series motor is 60 A when on full-load. If the load is adjusted to that this current decreases to 40-A, find the new torque expressed as a percentage of the full-load torque. The flux for a current of 40 A is 70% of that when current is 60 A. [46%]
5. A 4-pole, d.c. shunt motor has a flux per pole of 0.04 Wb and the armature is lap-wound with 720 conductors. The shunt field resistance is 240 W and the armature resistance is 0.2 W. Brush contact drop is 1V per brush. Determine the speed of the machine when running (a) as a motor taking 60 A and (b) as a generator supplying 120 A. The terminal voltage in each case is 480 V. [972 r.p.m. ; 1055 r.p.m.]
6. A 25-kW shunt generator is delivering full output to 400-V bus-bars and is driven at 950 r.p.m. by belt drive. The belt breaks suddenly but the machine continues to run as a motor taking 25 kW from the bus-bars. At what speed does it run ? Take armature resistance including brush contact resistance as 0.5 W and field resistance as 160 W. [812.7 r.p.m.] (Elect. Technology, Andhra Univ. Apr. 1977)
7. A 4-pole, d.c. shunt motor has a wave-wound armature with 65 slots each containing 6 conductors. The flux per pole is 20 mWb and the armature has a resistance of 0.15 W. Calculate the motor speed when the machine is operating from a 250-V supply and taking a current of 60 A. [927 r.p.m.]
8. A 500-V, d.c. shunt motor has armature and field resistances of 0.5 W and 200 W respectively. When loaded and taking a total input of 25 kW, it runs at 400 r.p.m. Find the speed at which it must be driven as a shunt generator to supply a power output of 25 kW at a terminal voltage of 500 V. [442 r.p.m.]
9. A d.c. shunt motor runs at 900 r.p.m. from a 400 V supply when taking an armature current of 25 A. Calculate the speed at which it will run from a 230 V supply when taking an armature current of 15 A. The resistance of the armature circuit is 0.8 W. Assume the flux per pole at 230 V to have decreased to 75% of its value at 400 V. [595 r.p.m.]
10. A shunt machine connected to 250-A mains has an armature resistance of 0.12 W and field resistance of 100 W. Find the ratio of the speed of the machine as a generator to the speed as a motor, if line current is 80 A in both cases. [1.08] (Electrical Engineering-II, Bombay Univ. April. 1977, Madras Univ. Nov. 1978)
11. A 20-kW d.c. shunt generator delivering rated output at 1000 r.p.m. has a terminal voltage of 500 V. The armature resistance is 0.1 W, voltage drop per brush is 1 volt and the field resistance is 500 W.
Calculate the speed at which the machine will run as a motor taking an input of 20 kW from a 500 V d.c. supply. [976.1 r.p.m.] (Elect. Engg-I Bombay Univ. 1975)
12. A 4-pole, 250-V, d.c. shunt motor has a lap-connected armature with 960 conductors. The flux per pole is 2 ´ 10-2 Wb. Calculate the torque developed by the armature and the useful torque in newton-metre when the current taken by the motor is 30A. The armature resistance is 0.12 ohm and the field resistance is 125 W. The rotational losses amount to 825 W.
[85.5 N-m ; 75.3 N-m] (Electric Machinery-I, Madras Univ. Nov. 1979)

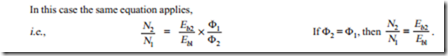















Comments
Post a Comment