D.c. Motor:Armature Torque of a Motor and Shaft Torque

By -

Armature Torque of a Motor

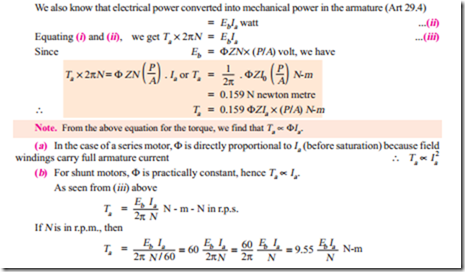
Let Ta be the torque developed by the armature of a motor running at N r.p.s. If Ta is in N/M, then power developed = Ta ´ 2p N watt

image

Shaft Torque

The whole of the armature torque, as calculated above, is not available for doing useful work, because a certain percentage of it is required for supplying iron and friction losses in the motor.

The torque which is available for doing useful work is known as shaft torque Tsh. It is so called because it is available at the shaft. The motor output is given by

image

Example 29.7. A 250-V, 4-pole, wave-wound d.c. series motor has 782 conductors on its armature. It has armature and series field resistance of 0.75 ohm. The motor takes a current of 40 A. Estimate its speed and gross torque developed if it has a flux per pole of 25 mWb.

image

image

image

image

Since, the terminal voltage is same in both the cases, shunt field current remains as 2.5 amp. With armature reaction is ignored, the flux/pole remains same. The e.m.f. then becomes proportional to the speed. If the generator must be driven at N r.p.m.

image

Example 29.15. The armature winding of a 200-V, 4-pole, series motor is lap-connected. There are 280 slots and each slot has 4 conductors. The current is 45 A and the flux per pole is 18 mWb. The field resistance is 0.3 W; the armature resistance 0.5 W and the iron and friction losses total 800 W. The pulley diameter is 0.41 m. Find the pull in newton at the rim of the pulley.

(Elect. Engg. AMIETE Sec. A. 1991)

image

Example 29.18. A 460-V, 55.95 kW, 750 r.p.m. shunt motor drives a load having a moment of inertia of 252.8 kg-m2. Find approximate time to attain full speed when starting from rest against full-load torque if starting current varies between 1.4 and 1.8 times full-load current.

Solution. Let us suppose that the starting current has a steady value of (1.4 + 1.8)/2 = 1.6 times full-load value.

image

image

Comments

Post a Comment

Popular posts from this blog

Total Loss in a D.C. Generator

By -
Image
Total Loss in a D.C. Generator The various losses occurring in a generator can be sub-divided as follows : where R a = resistance of armature and interpoles and series field winding etc. This loss is about 30 to 40% of full-load losses. Field copper loss. In the case of shunt generators, it is practically constant and I 2 R (or V I sh ). In the case of series generator, it is = I s e 2 R s e where R s e is resistance of the series field winding. This loss is about 20 to 30% of F.L. losses. (iii) The loss due to brush contact resistance. It is usually included in the armature copper loss. (b) Magnetic Losses (also known as iron or core losses), (i) hysteresis loss, W h µ B max f and ( ii ) eddy current loss, W e µ B max f These losses are practically constant for shunt and compound-wound generators, because in their case, field current is approximately constant. Both these losses total up to about 20 to 30% of F.L. losses. (c) Mechanica l Losses. These consist of :...
Read more

Transformer,three phase:Wye/Delta or Y/∆ Connection and Delta/Wye or ∆/Y Connection.

By -
Image
Wye/Delta or Y/∆  Connection The main use of this connection is at the substation end of the transmission line where the voltage is to be stepped down. The primary winding is Y -connected with grounded neutral as shown in Fig. 33.6. The ratio between the secondary and primary line voltage is 1/ 3 times the transformation ratio of each trans- former. There is a 30° shift between the primary and secondary line voltages which means that a Y - D transformer bank cannot be paralleled with either a Y - Y or a D - D bank. Also, third harmonic currents flows in the D to provide a sinusoidal flux. Delta/Wye or ∆/Y Connection This connection is generally employed where it is necessary to step up the voltage as for example, at the beginning of high tension transmission system. The connection is shown in Fig. 33.7. The neutral of the secondary is grounded for providing 3-phase 4-wire service. In recent years, this connection has gained considerable popularity because it can be used to s...
Read more

Testing of d.c.Machines:Brake Test

By -
Image
Brake Test It is a direct method and consists of applying a brake to a water-cooled pulley mounted on the motor shaft as shown in Fig. 31.1. The brake band is fixed with the help of wooden blocks gripping the pulley. One end of the band is fixed to earth via a spring balance S and the other is connected to a suspended weight W 1. The motor is running and the load on the motor is adjusted till it carries its full load current. The simple brake test described above can be used for small motors only, because in the case of large motors, it is difficult to dissipate the large amount of heat generated at the brake. Another simple method of measuring motor output is by the use of poney brake one form of which is shown in Fig. 31.2 ( a ). A rope is wound round the pulley and its two ends are attached to two spring balances S 1 and S 2. The tension of the rope can be adjusted with the help of swivels. Obviously, the force acting tangentially on the pulley is equal to the differ ence ...
Read more